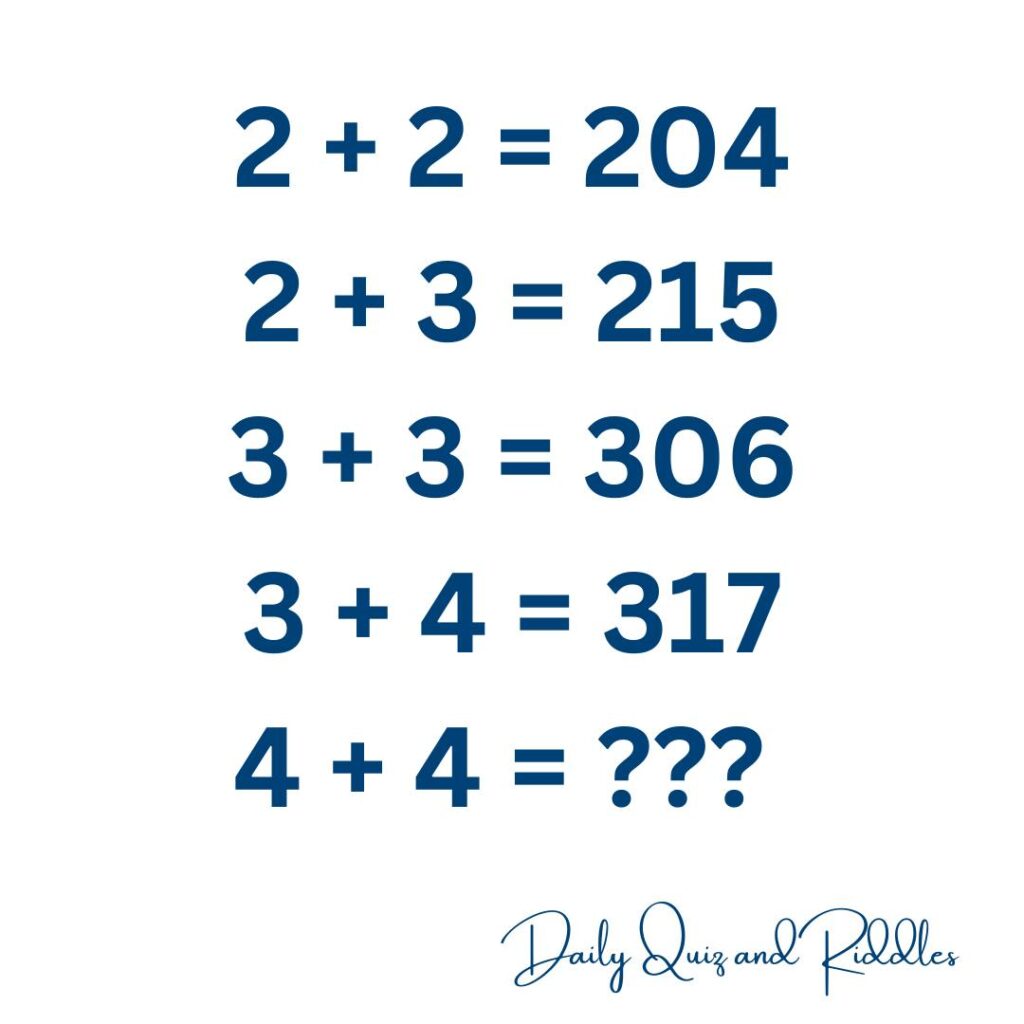Question:
Find the value of 4+4.
Answer:
408
Explanation
Welcome to this intriguing puzzle that may initially appear like a math challenge, but in reality, it’s all about deciphering patterns and applying logical reasoning. Let’s embark on this exciting journey of pattern recognition!
In this series of equations, we are given different additions, and our task is to find the value of 4 + 4. Let’s analyze the given equations to unveil the hidden pattern.
In the first equation, 2 + 2 = 204, we notice that the first number, 2, remains the same in each equation.
Similarly, in the second, third, and fourth equations, the first numbers 2,3,3 respectively maintained their first positions in all the answers. We can thus conclude that the first number in our problem is 4! Now we have 4+4 = 4??
Going to the second number, we realize that the second number is either 1 or 0, depending on whether the sum of the two numbers is even or odd. If the sum is even, the second number is 0. If the sum is odd, the second number is 1.
Applying this pattern to the given equations, we can deduce the following:
- 2 + 2 = 215 (2 + 2 = 4, even sum, second number is 0)
- 2 + 3 = 215 (2 + 3 = 5, odd sum, second number is 1)
- 3 + 3 = 306 (3 + 3 = 6, even sum, second number is 0)
- 3 + 4 = 317 (3 + 4 = 7, odd sum, second number is 1)
Now, let’s solve the final equation, 4 + 4. The sum of 4 + 4 is 8, an even number. Following the pattern, we conclude that the second number is 0. Therefore, the value of 4 + 4 = 40?.
Now finally, the third term is the easiest. We realize that the third number is simply the addition of both numbers.
- 2 + 2 = 215 (2 + 2 = 4, third number is 4)
- 2 + 3 = 215 (2 + 3 = 5, third number is 5)
- 3 + 3 = 306 (3 + 3 = 6, third number is 6)
- 3 + 4 = 317 (3 + 4 = 7, third number is 7)
Applying these patterns finally brings us to the final value of 4+4 = 408, which is the solution to our puzzle!
Isn’t it fascinating how patterns can unlock the secrets behind seemingly complex puzzles? It’s all about observing the relationships between numbers and applying logical reasoning to arrive at the solution.